Fracture Mechanics: Part Two
Abstract
Linear elastic fracture mechanics (LEFM) principles are used to relate the stress magnitude and distribution near the crack tip to (1) remote stresses applied to the cracked component, (2) the crack size and shape, and (3) the material properties of the cracked component.
This article gives a brief historical overview of Linear Elastic Fracture Mechanics.
Historical Overview
In the 1920s, Griffith formulated the concept that a crack in a component will propagate if the total energy of the system is lowered with crack propagation. That is, if the change in elastic strain energy due to crack extension is larger than the energy required to create new crack surfaces, crack propagation will occur.
Griffith's theory was developed for brittle materials. In the 1940s, Irwin extended the theory for ductile materials. He postulated that the energy due to plastic deformation must be added to the surface energy associated with the creation of new crack surfaces. He recognized that for ductile materials, the surface energy term is often negligible compared to the energy associated with plastic deformation.
Further, he defined a quantity, G, the strain energy release rate or "crack driving force," which is the total energy absorbed during cracking per unit increase in crack length and per unit thickness.
In the mid-1950s, Irwin made another significant contribution. He showed that the local stresses near the crack tip are of the general form
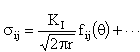 |
(1) |
where r and q are cylindrical coordinates of a point with respect to the crack tip (see Fig. 2) and K is the stress intensity factor.
He further showed that the energy approach (the "G" approach above) is equivalent to the stress intensity approach and that crack propagation occurs when a critical strain energy release rate, G, (or in terms of a critical stress intensity, Kc) is achieved.
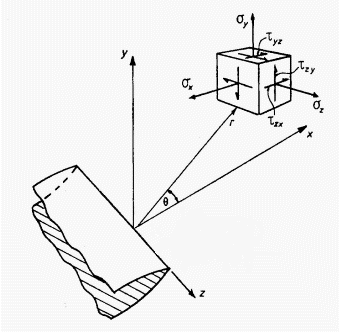 Figure 2. Location of local stresses near a crack tip in cylindrical
Figure 2. Location of local stresses near a crack tip in cylindrical
coordinates
LEFM Assumptions
Linear elastic fracture mechanics (LEFM) is based on the application of the theory of elasticity to bodies containing cracks or defects. The assumptions used in elasticity are also inherent in the theory of LEFM: small displacements and general linearity between stresses and strains.
The general form of the LEFM equations is given in Eq. 1. As seen, a singularity exists such that as r, the distance from the crack tip, tends toward zero, the stresses go to infinity. Since materials plastically deform as the yield stress is exceeded, a plastic zone will form near the crack tip.
The basis of LEFM remains valid, though, if this region of plasticity remains small in relation to the overall dimensions of the crack and cracked body.
Access Fracture Mechanics Properties of Thousands of Materials Now!
Total Materia Horizon includes a unique collection of fracture mechanics properties such as K1C, KC, crack growth and Paris law parameters, for thousands of metal alloys and heat treatments.
Get a FREE test account at Total Materia Horizon and join a community of over 500,000 users from more than 120 countries.