True Stress - True Strain Curve: Part Four

---

Introduction to Flow Curves and Material Constants

The flow curve of metals in the uniform plastic deformation region can be expressed through a simple power curve relation:

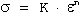      ...(10)

where n represents the strain-hardening exponent and K is the strength coefficient. When plotted on a log-log scale, true stress and true strain up to maximum load will yield a straight line if the data satisfies Equation (10).

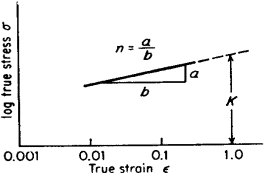
Figure 2. Log/log plot of true stress-strain curve

Understanding Strain-Hardening Parameters

The linear slope of this logarithmic plot equals n, while K represents the true stress at ε = 1.0 (corresponding to θ = 0.63). The strain-hardening exponent ranges from n = 0 (indicating a perfectly plastic solid) to n = 1 (representing an elastic solid). Most metals exhibit n values between 0.10 and 0.50, see Table 1.

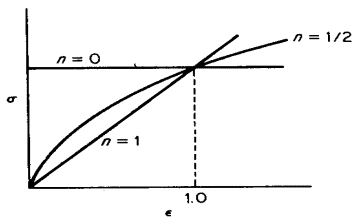
Figure 3. Various forms of power curve s=K* e ⁿ

Table 1. Values for n and K for metals at room temperature

Metal	Condition	n	K, psi
0,05% C steel	Annealed	0,26	77000
SAE 4340 steel	Annealed	0,15	93000
0,60% C steel	Quenched and tempered 1000oF	0,10	228000
0,60% C steel	Quenched and tempered 1300oF	0,19	178000
Copper	Annealed	0,54	46400
70/30 brass	Annealed	0,49	130000

Material-Specific Applications and Variations

The strain-hardening rate (dσ/dε) differs from the strain-hardening exponent. This relationship can be expressed as:

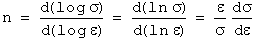
or

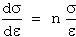      ...(11)

Advanced Equations and Deviations

Equation (10), while useful, is not universally applicable. Significant deviations frequently occur, particularly at low strains (10⁻³) or high strains (ε»1.0). These deviations manifest in several important ways:

Common Deviations in Log-Log Plots

When plotting Equation (10) on a log-log scale, one frequent deviation results in two distinct straight lines with different slopes. In cases where data doesn't conform to Equation (10), it may instead follow the relationship:

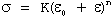      ...(12)

Here, Datsko's research demonstrates that ε₀ represents the material's strain hardening accumulated before the tension test.

The Ludwig Equation

A significant modification to the basic relationship is the Ludwig equation:

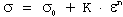      ...(13)

In this equation, σ₀ represents the yield stress, while K and n maintain their original definitions from Equation (10). The Ludwig equation offers a more accurate representation since it accounts for non-zero stress at zero strain, addressing a key limitation of Equation (10).

Special Cases and Material-Specific Variations

Morrison's work provides a method for determining σ₀ through the intersection of the strain-hardening point and the elastic modulus line. For specific materials like austenitic stainless steel, which show marked deviations at low strains, the relationship can be expressed as:

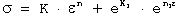
where:

• e_K approximates the proportional limit
• n₁ represents the slope of stress deviation from Equation (10) plotted against ε

Plastic Strain Considerations

For all equations (10-13), the true strain term should properly represent the plastic strain:

e_p= e_total- e_E= e_total- s/E